isaac newton biography timeline

• History Classics
• Your Profile
• Find History on Facebook (Opens in a new window)
• Find History on Twitter (Opens in a new window)
• Find History on YouTube (Opens in a new window)
• Find History on Instagram (Opens in a new window)
• Find History on TikTok (Opens in a new window)
• This Day In History
• History Podcasts
• History Vault

Isaac Newton

By: History.com Editors

Updated: October 16, 2023 | Original: March 10, 2015

Isaac Newton is best know for his theory about the law of gravity, but his “Principia Mathematica” (1686) with its three laws of motion greatly influenced the Enlightenment in Europe. Born in 1643 in Woolsthorpe, England, Sir Isaac Newton began developing his theories on light, calculus and celestial mechanics while on break from Cambridge University. 

Years of research culminated with the 1687 publication of “Principia,” a landmark work that established the universal laws of motion and gravity. Newton’s second major book, “Opticks,” detailed his experiments to determine the properties of light. Also a student of Biblical history and alchemy, the famed scientist served as president of the Royal Society of London and master of England’s Royal Mint until his death in 1727.

Isaac Newton: Early Life and Education

Isaac Newton was born on January 4, 1643, in Woolsthorpe, Lincolnshire, England. The son of a farmer who died three months before he was born, Newton spent most of his early years with his maternal grandmother after his mother remarried. His education was interrupted by a failed attempt to turn him into a farmer, and he attended the King’s School in Grantham before enrolling at the University of Cambridge’s Trinity College in 1661.

Newton studied a classical curriculum at Cambridge, but he became fascinated by the works of modern philosophers such as René Descartes, even devoting a set of notes to his outside readings he titled “Quaestiones Quaedam Philosophicae” (“Certain Philosophical Questions”). When the Great Plague shuttered Cambridge in 1665, Newton returned home and began formulating his theories on calculus, light and color, his farm the setting for the supposed falling apple that inspired his work on gravity.

Isaac Newton’s Telescope and Studies on Light

Newton returned to Cambridge in 1667 and was elected a minor fellow. He constructed the first reflecting telescope in 1668, and the following year he received his Master of Arts degree and took over as Cambridge’s Lucasian Professor of Mathematics. Asked to give a demonstration of his telescope to the Royal Society of London in 1671, he was elected to the Royal Society the following year and published his notes on optics for his peers.

Through his experiments with refraction, Newton determined that white light was a composite of all the colors on the spectrum, and he asserted that light was composed of particles instead of waves. His methods drew sharp rebuke from established Society member Robert Hooke, who was unsparing again with Newton’s follow-up paper in 1675. 

Known for his temperamental defense of his work, Newton engaged in heated correspondence with Hooke before suffering a nervous breakdown and withdrawing from the public eye in 1678. In the following years, he returned to his earlier studies on the forces governing gravity and dabbled in alchemy.

Isaac Newton and the Law of Gravity

In 1684, English astronomer Edmund Halley paid a visit to the secluded Newton. Upon learning that Newton had mathematically worked out the elliptical paths of celestial bodies, Halley urged him to organize his notes. 

The result was the 1687 publication of “Philosophiae Naturalis Principia Mathematica” (Mathematical Principles of Natural Philosophy), which established the three laws of motion and the law of universal gravity. Newton’s three laws of motion state that (1) Every object in a state of uniform motion will remain in that state of motion unless an external force acts on it; (2) Force equals mass times acceleration: F=MA and (3) For every action there is an equal and opposite reaction.

“Principia” propelled Newton to stardom in intellectual circles, eventually earning universal acclaim as one of the most important works of modern science. His work was a foundational part of the European Enlightenment .

With his newfound influence, Newton opposed the attempts of King James II to reinstitute Catholic teachings at English Universities. King James II was replaced by his protestant daughter Mary and her husband William of Orange as part of the Glorious Revolution of 1688, and Newton was elected to represent Cambridge in Parliament in 1689. 

Newton moved to London permanently after being named warden of the Royal Mint in 1696, earning a promotion to master of the Mint three years later. Determined to prove his position wasn’t merely symbolic, Newton moved the pound sterling from the silver to the gold standard and sought to punish counterfeiters.

The death of Hooke in 1703 allowed Newton to take over as president of the Royal Society, and the following year he published his second major work, “Opticks.” Composed largely from his earlier notes on the subject, the book detailed Newton’s painstaking experiments with refraction and the color spectrum, closing with his ruminations on such matters as energy and electricity. In 1705, he was knighted by Queen Anne of England.

Isaac Newton: Founder of Calculus?

Around this time, the debate over Newton’s claims to originating the field of calculus exploded into a nasty dispute. Newton had developed his concept of “fluxions” (differentials) in the mid 1660s to account for celestial orbits, though there was no public record of his work. 

In the meantime, German mathematician Gottfried Leibniz formulated his own mathematical theories and published them in 1684. As president of the Royal Society, Newton oversaw an investigation that ruled his work to be the founding basis of the field, but the debate continued even after Leibniz’s death in 1716. Researchers later concluded that both men likely arrived at their conclusions independent of one another.

Death of Isaac Newton

Newton was also an ardent student of history and religious doctrines, and his writings on those subjects were compiled into multiple books that were published posthumously. Having never married, Newton spent his later years living with his niece at Cranbury Park near Winchester, England. He died in his sleep on March 31, 1727, and was buried in Westminster Abbey .

A giant even among the brilliant minds that drove the Scientific Revolution, Newton is remembered as a transformative scholar, inventor and writer. He eradicated any doubts about the heliocentric model of the universe by establishing celestial mechanics, his precise methodology giving birth to what is known as the scientific method. Although his theories of space-time and gravity eventually gave way to those of Albert Einstein , his work remains the bedrock on which modern physics was built.

Isaac Newton Quotes

• “If I have seen further it is by standing on the shoulders of Giants.”
• “I can calculate the motion of heavenly bodies but not the madness of people.”
• “What we know is a drop, what we don't know is an ocean.”
• “Gravity explains the motions of the planets, but it cannot explain who sets the planets in motion.”
• “No great discovery was ever made without a bold guess.”

HISTORY Vault: Sir Isaac Newton: Gravity of Genius

Explore the life of Sir Isaac Newton, who laid the foundations for calculus and defined the laws of gravity.

Sign up for Inside History

Get HISTORY’s most fascinating stories delivered to your inbox three times a week.

By submitting your information, you agree to receive emails from HISTORY and A+E Networks. You can opt out at any time. You must be 16 years or older and a resident of the United States.

More details : Privacy Notice | Terms of Use | Contact Us

• Humanities ›
• History & Culture ›
• Inventions ›
• Famous Inventors ›

Biography of Isaac Newton, Mathematician and Scientist

Print Collector/Getty Images

• Famous Inventors
• Famous Inventions
• Patents & Trademarks
• Invention Timelines
• Computers & The Internet
• American History
• African American History
• African History
• Ancient History and Culture
• Asian History
• European History
• Latin American History
• Medieval & Renaissance History
• Military History
• The 20th Century
• Women's History

Sir Isaac Newton (Jan. 4, 1643–March 31, 1727) was a superstar of physics, math, and astronomy even in his own time. He occupied the chair of Lucasian Professor of Mathematics at the University of Cambridge in England, the same role later filled, centuries later, by Stephen Hawking . Newton conceived of several laws of motion , influential mathematical principals which, to this day, scientists use to explain how the universe works.

Fast Facts: Sir Isaac Newton

• Known For : Developed laws that explain how the universe works
• Born : Jan. 4, 1643 in Lincolnshire, England
• Parents : Isaac Newton, Hannah Ayscough
• Died : March 20, 1727 in Middlesex, England
• Education : Trinity College, Cambridge (B.A., 1665)
• Published Works : De Analysi per Aequationes Numero Terminorum Infinitas (1669, published 1711), Philosophiae Naturalis Principia Mathematica (1687), Opticks (1704)
• Awards and Honors : Fellowship of the Royal Society (1672), Knight Bachelor (1705)
• Notable Quote : "If I have seen further than others, it is by standing upon the shoulders of giants."

Early Years and Influences

Newton was born in 1642 in a manor house in Lincolnshire, England. His father had died two months before his birth. When Newton was 3 his mother remarried and he remained with his grandmother. He was not interested in the family farm, so he was sent to Cambridge University to study.

Newton was born just a short time after the death of  Galileo , one of the greatest scientists of all time. Galileo had proved that the planets revolve around the sun, not the earth as people thought at the time. Newton was very interested in the discoveries of Galileo and others. Newton thought the universe worked like a machine and that a few simple laws governed it. Like Galileo, he realized that mathematics was the way to explain and prove those laws.

Laws of Motion

Newton formulated laws of motion and gravitation. These laws are math formulas that explain how objects move when a force acts on them. Newton published his most famous book, "Principia," in 1687 while he was a mathematics professor at Trinity College in Cambridge. In "Principia," Newton explained three basic laws that govern the way objects move. He also described his theory of gravity, the force that causes things to fall down. Newton then used his laws to show that the planets revolve around the suns in orbits that are oval, not round.

The three laws are often called Newton’s Laws. The first law states that an object that is not being pushed or pulled by some force will stay still or will keep moving in a straight line at a steady speed. For example, if someone is riding a bike and jumps off before the bike is stopped, what happens? The bike continues on until it falls over. The tendency of an object to remain still or keep moving in a straight line at a steady speed is called inertia.

The second law explains how a force acts on an object. An object accelerates in the direction the force is moving it. If someone gets on a bike and pushes the pedals forward, the bike will begin to move. If someone gives the bike a push from behind, the bike will speed up. If the rider pushes back on the pedals, the bike will slow down. If the rider turns the handlebars, the bike will change direction.

The third law states that if an object is pushed or pulled, it will push or pull equally in the opposite direction. If someone lifts a heavy box, they use force to push it up. The box is heavy because it is producing an equal force downward on the lifter’s arms. The weight is transferred through the lifter’s legs to the floor. The floor also presses upward with an equal force. If the floor pushed back with less force, the person lifting the box would fall through the floor. If it pushed back with more force, the lifter would fly up in the air.

Importance of Gravity

When most people think of Newton, they think of him sitting under an apple tree observing an apple fall to the ground. When he saw the apple fall, Newton began to think about a specific kind of motion called gravity. Newton understood that gravity was a force of attraction between two objects. He also understood that an object with more matter or mass exerted the greater force or pulled smaller objects toward it. That meant that the large mass of the Earth pulled objects toward it. That is why the apple fell down instead of up and why people don’t float in the air.

He also thought that maybe gravity was not just limited to the Earth and the objects on the earth. What if gravity extended to the Moon and beyond? Newton calculated the force needed to keep the Moon moving around the earth. Then he compared it with the force that made the apple fall downward. After allowing for the fact that the Moon is much farther from the Earth and has a much greater mass, he discovered that the forces were the same and that the Moon is also held in orbit around Earth by the pull of earth’s gravity.

Disputes in Later Years and Death

Newton moved to London in 1696 to accept the position of warden of the Royal Mint. For many years afterward, he argued with Robert Hooke over who had actually discovered the connection between elliptical orbits and the inverse square law, a dispute that ended only with Hooke's death in 1703.

In 1705, Queen Anne bestowed a knighthood upon Newton, and thereafter he was known as Sir Isaac Newton. He continued his work, particularly in mathematics. This led to another dispute in 1709, this time with German mathematician Gottfried Leibniz. They both quarreled over which of them had invented calculus.

One reason for Newton's disputes with other scientists was his overwhelming fear of criticism, which led him to write, but then postpone publication of, his brilliant articles until after another scientist created similar work. Besides his earlier writings, "De Analysi" (which didn't see publication until 1711) and "Principia" (published in 1687), Newton's publications included "Optics" (published in 1704), "The Universal Arithmetic" (published in 1707), the "Lectiones Opticae" (published in 1729), the "Method of Fluxions" (published in 1736), and the "Geometrica Analytica" (printed in 1779).

On March 20, 1727, Newton died near London. He was buried in Westminster Abbey, the first scientist to receive this honor. 

Newton’s calculations changed the way people understood the universe. Prior to Newton, no one had been able to explain why the planets stayed in their orbits. What held them in place? People had thought that the planets were held in place by an invisible shield. Newton proved that they were held in place by the sun’s gravity and that the force of gravity was affected by distance and mass. While he was not the first person to understand that the orbit of a planet was elongated like an oval, he was the first to explain how it worked.

• “Isaac Newton's Life.”  Isaac Newton Institute for Mathematical Sciences.
• “ Isaac Newton Quotes. ”  BrainyQuote , Xplore.
• “ Sir Isaac Newton. ”  StarChild , NASA.
• Biography of Christiaan Huygens, Prolific Scientist
• Women in Mathematics History
• Biography of Joseph Louis Lagrange, Mathematician
• Biography of Galileo Galilei, Renaissance Philosopher and Inventor
• James Clerk Maxwell, Master of Electromagnetism
• Biography of Alan Turing, Code-Breaking Computer Scientist
• Biography of Ada Lovelace, First Computer Programmer
• The History of Tablet Compters
• Biography of Johannes Kepler, Pioneering German Astronomer
• Biography of Leonardo Pisano Fibonacci, Noted Italian Mathematician
• Biography of Charles Babbage, Mathematician and Computer Pioneer
• John Napier - Napier's Bones
• Biography of Srinivasa Ramanujan, Mathematical Genius
• Biography of John Napier, Scottish Mathematician
• Leonhard Euler, Mathematician: His Life and Work
• Biography of John Lee Love, Portable Pencil Sharpener Inventor

• Introduction to the Texts
• Mathematical
• His Notebooks
• by category
• His Life & Work at a Glance
• His Personal Life
• 18th Century
• 19th Century
• The Portsmouth Papers
• The Sotheby Sale
• Newton-related Papers of John Maynard Keynes
• Other Attempts to Publish Newton's Papers
• About Newton's Library
• Books in Newton's Library
• Bibliography
• The Newton Project
• Staff and Editorial Board
• Tagging & Transcription Guidelines
• Acknowledgments

Newton’s Life and Work at a Glance

The following tabular summary of Newton’s life and work does not pretend to be a comprehensive biography. It simply offers a quick and easy reference guide to the principal milestones in Newton’s personal and professional development, and correlates them with contemporary events and publications that influenced him.

For those wanting more detailed and nuanced accounts of Newton’s life and the various aspects of his thought, there is a wealth of material available online and in print. It would be impossible to provide an exhaustive list of such resources, but most of the best examples are listed on our Links page (for online material) and our Bibliography (for books and articles in print).

Note on dates: During Newton’s lifetime, two calendars were in use in Europe: the ‘Julian’ or ‘Old Style’ in Britain and parts of Eastern Europe, and the more accurate ‘Gregorian’ or ‘New Style’ elsewhere. The difference between them lay in their attitude to leap years. At Newton’s birth, Gregorian dates were ten days ahead of Julian dates: thus Newton was born on Christmas Day 1642 by the Julian calendar but on 4 January 1643 by the Gregorian. On either 19 February/1 March 1700 or 29 February/11 March 1700 (depending on which calendar is used to measure the gap), this discrepancy rose to eleven days, because there was no 29 February 1700 in the Gregorian calendar. Since some reference sources use one calendar, some the other, and some a mixture of both, this can cause considerable confusion. In the interests of clarifying apparent discrepancies with other sources, both options are given here wherever a particular date is specified.

Matters are further complicated by the contemporary English habit of regarding the year as beginning on 25 March. It is here regarded as beginning on 1 January, but notes are added where this may lead to confusion (for instance, the Complete Works of Joseph Mede are dated 1664 but were in fact published in the early months of what we now call 1665).

© 2024 The Newton Project

Professor Rob Iliffe Director, AHRC Newton Papers Project

Scott Mandelbrote, Fellow & Perne librarian, Peterhouse, Cambridge

Faculty of History, George Street, Oxford, OX1 2RL - [email protected]

Privacy Statement

 MacTutor

Isaac newton.

Threatening my father and mother Smith to burn them and the house over them.

... setting my heart on money, learning, and pleasure more than Thee ...

... changed his mind when he read that parallelograms upon the same base and between the same parallels are equal.

Thus Wallis doth it, but it may be done thus ...

[ Newton ] brought me the other day some papers, wherein he set down methods of calculating the dimensions of magnitudes like that of Mr Mercator concerning the hyperbola, but very general; as also of resolving equations; which I suppose will please you; and I shall send you them by the next.

... having no more acquaintance with him I did not think it becoming to urge him to communicate anything.

• investigations of the colours of thin sheets
• 'Newton's rings' and
• diffraction of light.

... that the Attraction always is in a duplicate proportion to the Distance from the Center Reciprocall ...

After his 1679 correspondence with Hooke , Newton, by his own account, found a proof that Kepler's areal law was a consequence of centripetal forces, and he also showed that if the orbital curve is an ellipse under the action of central forces then the radial dependence of the force is inverse square with the distance from the centre.

... asked Newton what orbit a body followed under an inverse square force, and Newton replied immediately that it would be an ellipse. However in 'De Motu..' he only gave a proof of the converse theorem that if the orbit is an ellipse the force is inverse square. The proof that inverse square forces imply conic section orbits is sketched in Cor. 1 to Prop. 13 in Book 1 of the second and third editions of the 'Principia', but not in the first edition.

... all matter attracts all other matter with a force proportional to the product of their masses and inversely proportional to the square of the distance between them.

Be courageous and steady to the Laws and you cannot fail.

Newton was of the most fearful, cautious and suspicious temper that I ever knew.

References ( show )

• I B Cohen, Biography in Dictionary of Scientific Biography ( New York 1970 - 1990) . See THIS LINK .
• Biography in Encyclopaedia Britannica. http://www.britannica.com/biography/Isaac-Newton
• E N da C Andrade, Isaac Newton ( New York, N. Y., 1950 , London, 1954) .
• Z Bechler, Newton's physics and the conceptual structure of the scientific revolution ( Dordrecht, 1991) .
• D Brewster, Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton (1855 , reprinted 1965) (2 volumes ) .
• G Castelnuovo, Le origini del calcolo infinitesimale nell'era moderna, con scritti di Newton, Leibniz, Torricelli ( Milan, 1962) .
• S Chandrasekhar, Newton's 'Principia' for the common reader ( New York, 1995) .
• G E Christianson, In the Presence of the Creator: Isaac Newton and His Times (1984) .
• I B Cohen, Introduction to Newton's 'Principia' ( Cambridge, Mass., 1971) .
• F Dessauer, Weltfahrt der Erkenntnis, Leben und Werk Isaac Newtons ( Zürich, 1945) .
• B J T Dobbs, and M C Jacob, Newton and the culture of Newtonianism. The Control of Nature ( Atlantic Highlands, NJ, 1995) .
• J Fauvel ( ed. ) , Let Newton Be! ( New York, 1988) .
• J O Fleckenstein, Die hermetische Tradition in der Kosmologie Newtons. Newton and the Enlightenment, Vistas Astronom. 22 (4) (1978) , 461 - 470 (1979) .
• F de Gandt, Force and geometry in Newton's 'Principia' ( Princeton, NJ, 1995) .
• D Gjertsen, The Newton Handbook ( London, 1986) .
• A R Hall, Isaac Newton, Adventurer in Thought ( Oxford, 1992) .
• J W Herivel, The background to Newton's 'Principia' : A study of Newton's dynamical researches in the years 1664 - 84 ( Oxford, 1965) .
• V Kartsev, Newton ( Russian ) , The Lives of Remarkable People. Biography Series ( Moscow, 1987) .
• J E McGuire and M Tamny, Certain philosophical questions : Newton's Trinity notebook ( Cambridge-New York, 1983) .
• D B Meli, Equivalence and priority : Newton versus Leibniz. Including Leibniz's unpublished manuscripts on the 'Principia' ( New York, 1993) .
• F Rosenberger, Isaac Newton und seine Physikalischen Principien ( Darmstadt, 1987) .
• H W Turnbull, The Mathematical Discoveries of Newton ( London, 1945) .
• R S Westfall, Never at Rest: A Biography of Isaac Newton (1990) .
• R S Westfall, The Life of Isaac Newton ( Cambridge, 1993) .
• H Wussing, Newton, in H Wussing and W Arnold, Biographien bedeutender Mathematiker ( Berlin, 1983) .
• J Agassi, The ideological import of Newton, Vistas Astronom. 22 (4) (1978) , 419 - 430 .
• E J Aiton, The solution of the inverse-problem of central forces in Newton's 'Principia', Arch. Internat. Hist. Sci. 38 (121) (1988) , 271 - 276 .
• E J Aiton, The contributions of Isaac Newton, Johann Bernoulli and Jakob Hermann to the inverse problem of central forces, in Der Ausbau des Calculus durch Leibniz und die Brüder Bernoulli ( Wiesbaden, 1989) , 48 - 58 .
• E J Aiton, The contributions of Newton, Bernoulli and Euler to the theory of the tides, Ann. of Sci. 11 (1956) , 206 - 223 .
• E N da C Andrade, Newton and the science of his age, Proc. Roy. Soc. London. Ser. A 181 (1943) , 227 - 243 .
• S Aoki, The moon-test in Newton's 'Principia' : accuracy of inverse-square law of universal gravitation, Arch. Hist. Exact Sci. 44 (2) (1992) , 147 - 190 .
• V I Arnol'd, and V A Vasil'ev, Newton's 'Principia' read 300 years later, Notices Amer. Math. Soc. 36 (9) (1989) , 1148 - 1154 .
• R T W Arthur, Newton's fluxions and equably flowing time, Stud. Hist. Philos. Sci. 26 (2) (1995) , 323 - 351 .
• K A Baird, Some influences upon the young Isaac Newton, Notes and Records Roy. Soc. London 41 (2) (1987) , 169 - 179 .
• M Bartolozzi and R Franci, A fragment of the history of algebra : Newton's rule on the number of imaginary roots in an algebraic equation ( Italian ) , Rend. Accad. Naz. Sci. XL Mem. Sci. Fis. Natur. (5) 14 (2 , II ) (1990) , 69 - 85 .
• P K Basu, Newton's physics in the context of his works on chemistry and alchemy, Indian J. Hist. Sci. 26 (3) (1991) , 283 - 305 .
• Z Bechler, Newton's ontology of the force of inertia, in The investigation of difficult things ( Cambridge, 1992) , 287 - 304 .
• Z Bechler, Newton's law of forces which are inversely as the mass : a suggested interpretation of his later efforts to normalise a mechanistic model of optical dispersion, Centaurus 18 (1973 / 74) , 184 - 222 .
• Z Bechler, 'A less agreeable matter' : the disagreeable case of Newton and achromatic refraction, British J. Hist. Sci. 8 (29) (1975) , 101 - 126 .
• Z Bechler, Newton's search for a mechanistic model of colour dispersion : a suggested interpretation, Arch. History Exact Sci. 11 (1973 / 74) , 1 - 37 .
• E T Bell, Newton after three centuries, Amer. Math. Monthly 49 (1942) , 553 - 575 .
• D Bertoloni Meli, Public claims, private worries : Newton's 'Principia' and Leibniz's theory of planetary motion, Stud. Hist. Philos. Sci. 22 (3) (1991) , 415 - 449 .
• T R Bingham, Newton and the development of the calculus, Pi Mu Epsilon J. 5 (1971) , 171 - 181 .
• J Blaquier, Sir Isaac Newton : The man and the mathematician ( Spanish ) , Anales Acad. Nac. Ci. Ex. Fis. Nat. Buenos Aires 12 (1947) , 9 - 32 .
• M Blay, Le traitement newtonien du mouvement des projectiles dans les milieux résistants, Rev. Histoire Sci. 40 (3 - 4) (1987) , 325 - 355 .
• M Blay, and G Barthélemy, Changements de repères chez Newton : le problème des deux corps dans les 'Principia', Arch. Internat. Hist. Sci. 34 (112) (1984) , 68 - 98 .
• C B Boyer, Newton as an originator of polar coördinates, Amer. Math. Monthly 56 (1949) , 73 - 78 .
• J B Brackenridge, The critical role of curvature in Newton's developing dynamics, in The investigation of difficult things ( Cambridge, 1992) , 231 - 260 .
• J B Brackenridge, Newton's unpublished dynamical principles : a study in simplicity, Ann. of Sci. 47 (1) (1990) , 3 - 31 .
• J B Brackenridge, Newton's mature dynamics and the 'Principia' : a simplified solution to the Kepler problem, Historia Math. 16 (1) (1989) , 36 - 45 .
• J B Brackenridge, Newton's mature dynamics : revolutionary or reactionary?, Ann. of Sci. 45 (5) (1988) , 451 - 476 .
• W J Broad, Sir Isaac Newton : mad as a hatter, Science 213 (4514) (1981) , 1341 - 1344 .
• P Casini, Newton's 'Principia' and the philosophers of the Enlightenment : Newton's 'Principia' and its legacy, Notes and Records Roy. Soc. London 42 (1) (1988) , 35 - 52 .
• P Casini, Newton : the classical scholia, Hist. of Sci. 22 (55 , 1) (1984) , 1 - 58 .
• M Cernohorsk'y, The rotation in Newton's wording of his first law of motion, in Isaac Newton's 'Philosophiae naturalis principia mathematica' ( Singapore, 1988) , 28 - 46 .
• S Chandrasekhar, Newton and Michelangelo, Current Sci. 67 (7) (1994) , 497 - 499 .
• S Chandrasekhar, On reading Newton's 'Principia' at age past eighty, Current Sci. 67 (7) (1994) , 495 - 496 .
• S Chandrasekhar, Shakespeare, Newton and Beethoven or patterns of creativity, Current Sci. 70 (9) (1996) , 810 - 822 .
• C A Chant, Isaac Newton : Born three hundred years ago, J. Roy. Astr. Soc. Canada 37 (1943) , 1 - 16 .
• C Christensen, Newton's method for resolving affected equations, College Math. J. 27 (5) (1996) , 330 - 340 .
• I B Cohen, Notes on Newton in the art and architecture of the Enlightenment, Vistas Astronom. 22 (4) (1978) , 523 - 537 .
• I B Cohen, Newton's 'System of the world' : some textual and bibliographical notes, Physis - Riv. Internaz. Storia Sci. 11 (1 - 4) (1969) , 152 - 166 .
• I B Cohen, Isaac Newton, the calculus of variations, and the design of ships, in For Dirk Struik ( Dordrecht, 1974) , 169 - 187 .
• I B Cohen, Newton's description of the reflecting telescope, Notes and Records Roy. Soc. London 47 (1) (1993) , 1 - 9 .
• A Cook, Edmond Halley and Newton's 'Principia', Notes and Records Roy. Soc. London 45 (2) (1991) , 129 - 138 .
• B P Copenhaver, Jewish theologies of space in the scientific revolution : Henry More, Joseph Raphson, Isaac Newton and their predecessors, Ann. of Sci. 37 (5) (1980) , 489 - 548 .
• P Costabel, Les 'Principia' de Newton et leurs colonnes d'Hercule, Rev. Histoire Sci. 40 (3 - 4) (1987) , 251 - 271 .
• G V Coyne, Newton's controversy with Leibniz over the invention of the calculus, in Newton and the new direction in science ( Vatican City, 1988) , 109 - 115 .
• J T Cushing, Kepler's laws and universal gravitation in Newton's 'Principia', Amer. J. Phys. 50 (7) (1982) , 617 - 628 .
• S D'Agostino, On the problem of the redundancy of absolute motion in Newton's 'Principia', Physis - Riv. Internaz. Storia Sci. 25 (1) (1983) , 167 - 169 .
• S Débarbat, Newton, Halley et l'Observatoire de Paris, Rev. Histoire Sci. 39 (2) (1986) , 127 - 154 .
• E Dellian, Newton, die Trägheitskraft und die absolute Bewegung, Philos. Natur. 26 (2) (1989) , 192 - 201 .
• C Dilworth, Boyle, Hooke and Newton : some aspects of scientific collaboration, Rend. Accad. Naz. Sci. XL Mem. Sci. Fis. Natur. (5) 9 (1985) , 329 - 331 .
• R Dimitri'c, Sir Isaac Newton, Math. Intelligencer 13 (1) (1991) , 61 - 65 .
• B J T Dobbs, Newton as final cause and first mover, Isis 85 (4) (1994) , 633 - 643 .
• M J Duck, Newton and Goethe on colour : physical and physiological considerations, Ann. of Sci. 45 (5) (1988) , 507 - 519 .
• R Duda, Newton and the mathematical concept of space, in Isaac Newton's 'Philosophiae naturalis principia mathematica' ( Singapore, 1988) , 72 - 83 .
• R Dugas, Le troisième centenaire de Newton, Revue Sci. 86 (1948) , 111 - 114 .
• S J Dundon, Newton's 'mathematical way' in the 'De mundi systemate', Physis - Riv. Internaz. Storia Sci. 11 (1 - 4) (1969) , 195 - 204 .
• A W F Edwards, Is the frontispiece of 'Gulliver's travels' a likeness of Newton?, Notes and Records Roy. Soc. London 50 (2) (1996) , 191 - 194 .
• S B Engelsman, Orthogonaltrajektorien im Prioritätsstreit zwischen Leibniz und Newton, in 300 Jahre 'Nova methodus' von G W Leibniz (1684 - 1984) ( Wiesbaden, 1986) , 144 - 156 .
• H Erlichson, Huygens and Newton on the Problem of Circular Motion, Centaurus 37 (1994) , 210 - 229 .
• H Erlichson, The visualization of quadratures in the mystery of Corollary 3 to Proposition 41 of Newton's 'Principia', Historia Math. 21 (2) (1994) , 148 - 161 .
• H Erlichson, Newton's polygon model and the second order fallacy, Centaurus 35 (3 - 4) (1992) , 243 - 258 .
• H Erlichson, Newton and Hooke on centripetal force motion, Centaurus 35 (1) (1992) , 46 - 63 .
• H Erlichson, Newton's first inverse solutions, Centaurus 34 (4) (1991) , 345 - 366 .
• H Erlichson, Newton's solution to the equiangular spiral problem and a new solution using only the equiangular property, Historia Math. 19 (4) (1992) , 402 - 413 .
• H Erlichson, How Newton went from a mathematical model to a physical model for the problem of a first power resistive force, Centaurus 34 (3) (1991) , 272 - 283 .
• H Erlichson, Motive force and centripetal force in Newton's mechanics, Amer. J. Phys. 59 (9) (1991) , 842 - 849 .
• H Erlichson, Newton's 1679 / 80 solution of the constant gravity problem, Amer. J. Phys. 59 (8) (1991) , 728 - 733 .
• N Feather, Rutherford - Faraday - Newton, Notes and Records Roy. Soc. London 27 (1972) , 45 - 55 .
• M Feingold, Newton, Leibniz, and Barrow too : an attempt at a reinterpretation, Isis 84 (2) (1993) , 310 - 338 .
• M Fellgett, Some influences on the young Isaac Newton, Notes and Records Roy. Soc. London 42 (2) (1988) , 243 .
• E A Fellmann, Über eine Bemerkung von G. W. Leibniz zu einem Theorem in Newtons 'Principia Mathematica', Historia Math. 5 (3) (1978) , 267 - 273 .
• E A Fellmann, Newtons 'Principia', Jber. Deutsch. Math.-Verein. 77 (3) (1975 / 76) , 107 - 137 .
• A Ferguson, Newton and the 'Principia', Philos. Mag. (7) 33 (1942) , 871 - 888 .
• C Ferrini, On Newton's demonstration of Kepler's second law in Hegel's 'De orbitis planetarum' (1801) , Philos. Natur. 31 (1) (1994) , 150 - 170 .
• M Fierz and M Fierz, Zur Genauigkeit von Newtons Messung seiner Interferenzringe, Helv. Phys. Acta 67 (8) (1994) , 923 - 929 .
• K Figala, J Harrison and U Petzold, 'De scriptoribus chemicis' : sources for the establishment of Isaac Newton's ( al ) chemical library, in The investigation of difficult things ( Cambridge, 1992) , 135 - 179 .
• S R Filonovich, Experiment in I Newton's 'Principia' ( Russian ) , Voprosy Istor. Estestvoznan. i Tekhn. (4) (1987) , 57 - 67 .
• G Findlay Shirras, Newton, a study of a master mind, Arch. Internat. Hist. Sci. ( N.S. ) 4 (1951) , 897 - 914 .
• M A Finocchiaro, Newton's third rule of philosophizing : a rule for logic in historiography, Isis 65 (1974) , 66 - 73 .
• E G Forbes, Newton's science and the Newtonian philosophy, Vistas Astronom. 22 (4) (1978) , 413 - 418 .
• A Franklin and C Howson, Newton and Kepler, a Bayesian approach, Stud. Hist. Philos. Sci. 16 (4) (1985) , 379 - 385 .
• H C Freiesleben, Newton's quadrant for navigation, Vistas Astronom. 22 (4) (1978) , 515 - 522 .
• A Gabbey, Newton's 'Mathematical principles of natural philosophy' : a treatise on 'mechanics'?, in The investigation of difficult things ( Cambridge, 1992) , 305 - 322 .
• M Gagnon, Les arguments de Newton concernant l'existence du mouvement, de l'espace et du temps absolus, Dialogue 25 (4) (1986) , 629 - 662 .
• M Galuzzi, Some considerations about motion in a resisting medium in Newton's 'Principia', in Conference on the History of Mathematics ( Rende, 1991) , 169 - 189 .
• F de Gandt, Le style mathématique des 'Principia' de Newton, Études sur l'histoire du calcul infinitésimal, Rev. Histoire Sci. 39 (3) (1986) , 195 - 222 .
• F de Gandt, The mathematical style of Newton's 'Principia', Mathesis. Mathesis 6 (2) (1990) , 163 - 189 .
• J Gani, Newton on 'a question touching ye different odds upon certain given chances upon dice', Math. Sci. 7 (1) (1982) , 61 - 66 .
• J Gascoigne, The universities and the scientific revolution : the case of Newton and Restoration Cambridge, Hist. of Sci. 23 (62 , 4) (1985) , 391 - 434 .
• I A Gerasimov, Newton and celestial mechanics ( Russian ) , Istor.-Astronom. Issled. 20 (1988) , 39 - 55 .
• E Giusti, A comparison of infinitesimal calculus in Leibniz and Newton ( Italian ) , Rend. Sem. Mat. Univ. Politec. Torino 46 (1) (1988) , 1 - 29 .
• J L Greenberg, Isaac Newton and the problem of the Earth's shape, Arch. Hist. Exact Sci. 49 (4) (1996) , 371 - 391 .
• J L Greenberg, Isaac Newton et la théorie de la figure de la Terre, Rev. Histoire Sci. 40 (3 - 4) (1987) , 357 - 366 .
• A T Grigor'yan, Isaac Newton's work of genius ( Russian ) , Studies in the history of physics and mechanics 1987 'Nauka' ( Moscow, 1987) , 177 - 191 ; 245 - 246 .
• A T Grigor'yan and B G Kuznetsov, On the 250 th anniversary of the death of Newton ( Russian ) , Organon 14 (1978) , 263 - 274 .
• U Grigull, Das Newtonsche Abkühlungsgesetz : Bemerkungen zu einer Arbeit von Isaac Newton aus dem Jahre 1701 , Physis - Riv. Internaz. Storia Sci. 20 (1 - 4) (1978) , 213 - 235 .
• E Grosholz, Some uses of proportion in Newton's 'Principia', Book I : a case study in applied mathematics, Stud. Hist. Philos. Sci. 18 (2) (1987) , 209 - 220 .
• H Guerlac, 'Newton's mathematical way' : another look, British J. Hist. Sci. 17 (55 , 1) (1984) , 61 - 64 .
• H Guerlac, Can we date Newton's early optical experiments?, Isis 74 (271) (1983) , 74 - 80 .
• Z Hajduk, Isaac Newton's philosophy of nature, in Isaac Newton's 'Philosophiae naturalis principia mathematica' ( Singapore, 1988) , 96 - 112 .
• A R Hall, Newton and the absolutes : sources, in The investigation of difficult things ( Cambridge, 1992) , 261 - 285 .
• A R Hall, Beyond the fringe : diffraction as seen by Grimaldi, Fabri, Hooke and Newton, Notes and Records Roy. Soc. London 44 (1) (1990) , 13 - 23 .
• A R Hall, Further Newton correspondence, Notes and Records Roy. Soc. London 37 (1) (1982) , 7 - 34 .
• M B Hall, Newton and his theory of matter in the eighteenth century, Vistas Astronom. 22 (4) (1978) , 453 - 459 .
• A R Hall, Newton in France : a new view, Hist. of Sci. 13 (4) (1975) , 233 - 250 .
• A R Hall, Newton and his editors, Notes and Records Roy. Soc. London 29 (1974) , 29 - 52 .
• A R Hall, John Collins on Newton's telescope, Notes and Records Roy. Soc. London 49 (1) (1995) , 71 - 78 .
• F Hammer, Newtons Bedeutung für den Dialog zwischen Naturwissenschaft und Theologie, Philos. Natur. 20 (1) (1983) , 3 - 13 .
• P M Harman, Newton to Maxwell : the 'Principia' and British physics. Newton's 'Principia' and its legacy, Notes and Records Roy. Soc. London 42 (1) (1988) , 75 - 96 .
• J L Hawes, Newton's revival of the aether hypothesis and the explanation of gravitational attraction, Notes and Records Roy. Soc. London 23 (1968) , 200 - 212 .
• S W Hawking, Newton's 'Principia', in Three hundred years of gravitation ( Cambridge, 1987) , 1 - 4 .
• A Hayli and Ph Kerspern, Le grand tournant de la pensée newtonienne de 1688 - 1690 , Vistas Astronom. 22 (4) (1978) , 511 - 514 .
• J Hendry, Newton's theory of colour, Centaurus 23 (3) (1979 / 80) , 230 - 251 .
• J W Herivel, Newtonian studies. III : The originals of the two propositions discovered by Newton in December 1679 ?, Arch. Internat. Histoire Sci. 14 (1961) , 23 - 33 .
• J W Herivel, Sur les premières recherches de Newton en dynamique, Rev. Histoire Sci. Appl. 15 (1962) , 105 - 140 .
• J E Hofmann, Der junge Newton als Mathematiker (1665 - 1675) , Math.-Phys. Semesterber. 2 (1951) , 45 - 70 .
• S H Hollingdale, Towards the tercentenary of Newton's 'Principia', Bull. Inst. Math. Appl. 18 (9 - 10) (1982) , 178 - 183 .
• S H Hollingdale, On reading Newton's 'Opticks', Bull. Inst. Math. Appl. 17 (1) (1981) , 2 - 6 .
• S H Hollingdale, The apotheosis of Isaac Newton, Bull. Inst. Math. Appl. 14 (8 - 9) (1978) , 194 - 195 .
• M A Hoskin, Newton and Lambert, Vistas Astronom. 22 (4) (1978) , 483 - 484 .
• M Hoskin, Newton and the beginnings of stellar astronomy, in Newton and the new direction in science ( Vatican City, 1988) , 55 - 63 .
• R C Hovis, What can the history of mathematics learn from philosophy? A case study in Newton's presentation of the calculus, Philos. Math. (2) 4 (1) (1989) , 35 - 57 .
• M Hughes, Newton, Hermes and Berkeley, British J. Philos. Sci. 43 (1) (1992) , 1 - 19 .
• D W Hutchings, Isaac Newton, 1642 - 1727 , in Late seventeenth century scientists ( Oxford, 1969) , 158 - 183 .
• M de Icaza Herrera, Galileo, Bernoulli, Leibniz and Newton around the brachistochrone problem, Rev. Mexicana Fis. 40 (3) (1994) , 459 - 475 .
• T Ishigaki, Newton's 'Principia' from a logical point of view, Ann. Japan Assoc. Philos. Sci. 8 (4) (1994) , 221 - 236 .
• A Yu Ishlinskii, Inertia forces in Newton's world ( Russian ) , Priroda (3) (1989) , 66 - 74 .
• A Jacob, The metaphysical systems of Henry More and Isaac Newton, Philos. Natur. 29 (1) (1992) , 69 - 93 .
• W B Joyce and A Joyce, Descartes, Newton, and Snell's law, J. Opt. Soc. Amer. 66 (1) (1976) , 1 - 8 .
• R Kargon, Newton, Barrow and the hypothetical physics, Centaurus 11 (1) (1965 / 66) , 46 - 56 .
• P Kerszberg, The cosmological question in Newton's science, Osiris (2) 2 (1986) , 69 - 106 .
• M Keynes, The personality of Isaac Newton, Notes and Records of the Royal Society of London 49 (1995) , 1 - 56 .
• P V Kharlamov, The concept of force in Newton's mechanics ( Russian ) , Mekh. Tverd. Tela 20 (1988) , 46 - 67 ; 112 .
• C W Kilmister, The history of Newton's laws, Bull. Inst. Math. Appl. 17 (8 - 9) (1981) , 173 - 176 .
• V S Kirsanov, Newton and his epoch ( Russian ) , Voprosy Istor. Estestvoznan. i Tekhn. (1) (1993) , 16 - 18 .
• V S Kirsanov, The correspondence between Isaac Newton and Robert Hooke : 1679 - 80 ( Russian ) , Voprosy Istor. Estestvoznan. i Tekhn. (4) (1996) , 3 - 39 ; 173 .
• V S I Kirsanov, Newton's early ideas about gravity (1665 - 1669) ( Russian ) , Voprosy Istor. Estestvoznan. i Tekhn. (2) (1993) , 42 - 52 ; 172 .
• P Kitcher, Fluxions, limits, and infinite littlenesse : A study of Newton's presentation of the calculus, Isis 64 (221) (1973) , 33 - 49 .
• O Knudsen, A note of Newton's concept of force, Centaurus 9 (1963 / 1964) , 266 - 271 .
• N Kollerstrom, Newton's two 'moon-tests', British J. Hist. Sci. 24 (82 , 3) (1991) , 369 - 372 .
• N Kollerstrom and B D Yallop, Flamsteed's lunar data, 1692 - 95 , sent to Newton, J. Hist. Astronom. 26 (3) (1995) , 237 - 246 .
• A Koyré, Pour une édition critique des oeuvres de Newton, Rev. Hist. Sci. Appl. 8 (1955) , 19 - 37 .
• F D Kramar, Questions of the foundations of analysis in the works of Wallis and Newton ( Russian ) , Trudy Sem. MGU Istor. Mat. Istor.-Mat. Issledov. 1950 (3) (1950) , 486 - 508 .
• T M Kuk, On the question of Newton's physical concept of force ( Russian ) , Sketches on the history of mathematical physics 'Naukova Dumka' ( Kiev, 1985) , 80 - 83 ; 185 .
• L L Kul'vetsas, The content of the concept of force in Newton's mechanics ( Russian ) , in Studies in the history of physics and mechanics, 1990 'Nauka' ( Moscow, 1990) , 131 - 149 .
• B G Kuznecov, The teaching of Newton on relativity and absolute motion ( Russian ) , Izvestiya Akad. Nauk SSSR. Ser. Istor. Filos. 5 (1948) , 149 - 166 .
• T Lai, Did Newton renounce infinitesimals?, Historia Math. 2 (1975) , 127 - 136 .
• V P Lishevskii, The genius of the natural sciences ( on the occasion of the 350 th anniversary of the birth of Isaac Newton ) ( Russian ) , Vestnik Ross. Akad. Nauk 63 (1) (1993) , 33 - 37 .
• J E Littlewood, Newton and the attraction of a sphere, Math. Gaz. 32 (1948) , 179 - 181 .
• J A Lohne, Hooke versus Newton : An analysis of the documents in the case on free fall and planetary motion, Centaurus 7 (1960) , 6 - 52 .
• J A Lohne, Newton's table of refractive powers. Origins, accuracy, and influence, Sudhoffs Arch. 61 (3) (1977) , 229 - 247 .
• J A Lohne, Fermat, Newton, Leibniz und das anaklastische Problem, Nordisk Mat. Tidskr. 14 (1966) , 5 - 25 .
• J Marek, Newton's report 'New theory about light and colours' and its relation to results of his predecessors, Physis - Riv. Internaz. Storia Sci. 11 (1 - 4) (1969) , 390 - 407 .
• J E McGuire, Newton on place, time, and God : an unpublished source, British J. Hist. Sci. 11 (38 , 2) (1978) , 114 - 129 .
• J E McGuire and M Tamny, Newton's astronomical apprenticeship : notes of 1664 / 5 , Isis 76 (283) (1985) , 349 - 365 .
• F A Medvedev, Horn angles in the works of I Newton ( Russian ) , Istor.-Mat. Issled. 31 (1989) , 18 - 37 .
• A Michalik, Mathematical structure of nature in Newton's 'Definitions and Scholium', in Newton and the new direction in science ( Vatican City, 1988) , 265 - 269 .
• F Mignard, The theory of the figure of the Earth according to Newton and Huygens, Vistas Astronom. 30 (3 - 4) (1987) , 291 - 311 .
• M Miller, Newtons Differenzenmethode, Wiss. Z. Hochsch. Verkehrswes. Dresden 2 (3) (1954) , 1 - 13 .
• M Miller, Isaac Newton : Über die Analysis mit Hilfe unendlicher Reihen, Wiss. Z. Hochsch. Verkehrswes. Dresden 2 (2) (1954) , 1 - 16 .
• M Miller, Newton, Aufzahlung der Linien dritter Ordnung, Wiss. Z. Hochsch. Verkehrswes. Dresden 1 (1) (1953) , 5 - 32 .
• A A Mills, Newton's water clocks and the fluid mechanics of clepsydrae, Notes and Records Roy. Soc. London 37 (1) (1982) , 35 - 61 .
• A A Mills, Newton's prisms and his experiments on the spectrum, Notes and Records Roy. Soc. London 36 (1) (1981 / 82) , 13 - 36 .
• J D Moss, Newton and the Jesuits in the 'Philosophical transactions', in Newton and the new direction in science ( Vatican City, 1988) , 117 - 134 .
• H Nakajima, Two kinds of modification theory of light : some new observations on the Newton-Hooke controversy of 1672 concerning the nature of light, Ann. of Sci. 41 (3) (1984) , 261 - 278 .
• M Nauenberg, Huygens and Newton on Curvature and its applications to Dynamics, De zeventiende eeuw, jaargang 12 (1) (1996) , 215 - 234 .
• M Nauenberg, Newton's early computational method, Archive for the History of the Exact Science 46 (3) (1994) , 221 - 252 .
• M Nauenberg, Newton's Principia and Inverse square orbits, The College Mathematics Journal 25 (1994) , 213 - 222 .
• M Nauenberg, Newton's early computational method for dynamics, Arch. Hist. Exact Sci. 46 (3) (1994) , 221 - 252 .
• T Needham, Newton and the transmutation of force, Amer. Math. Monthly 100 (2) (1993) , 119 - 137 .
• J M Nicholas, Newton's extremal second law, Centaurus 22 (2) (1978 / 79) , 108 - 130 .
• C T O'Sullivan, Newton's laws of motion : some interpretations of the formalism, Amer. J. Phys. 48 (2) (1980) , 131 - 133 .
• M Panza, Eliminating time : Newton, Lagrange and the inverse problem of resisting motion ( Italian ) , in Conference on the History of Mathematics ( Rende, 1991) , 487 - 537 .
• J Peiffer, Leibniz, Newton et leurs disciples, Rev. Histoire Sci. 42 (3) (1989) , 303 - 312 .
• A Pérez de Laborda, Newtons Fluxionsrechnung im Vergleich zu Leibniz' Infinitesimalkalkül, in 300 Jahre 'Nova methodus' von G W Leibniz (1684 - 1984) ( Wiesbaden, 1986) , 239 - 257 .
• S Pierson, Two mathematics, two Gods : Newton and the second law, Perspect. Sci. 2 (2) (1994) , 231 - 253 .
• B Pourciau, Reading the master : Newton and the birth of celestial mechanics, Amer. Math. Monthly 104 (1) (1997) , 1 - 19 .
• B Pourciau, Newton's solution of the one-body problem, Arch. Hist. Exact Sci. 44 (2) (1992) , 125 - 146 .
• B H Pourciau, On Newton's proof that inverse-square orbits must be conics, Ann. of Sci. 48 (2) (1991) , 159 - 172 .
• A Prince, The phenomenalism of Newton and Boscovich : a comparative study, Synth. Philos. 4 (2) (1989) , 591 - 618 .
• T Retnadevi, The life and contributions of a mathematician whom I respect - Sir Isaac Newton, Menemui Mat. 5 (1) (1983) , 25 - 33 .
• V F Rickey, Isaac Newton : man, myth and mathematics, Mathesis. Mathesis 6 (2) (1990) , 119 - 162 .
• V F Rickey, Isaac Newton : man, myth, and mathematics, College Math. J. 18 (5) (1987) , 362 - 389 .
• G A J Rogers, The system of Locke and Newton, in Contemporary Newtonian research ( Dordrecht-Boston, Mass., 1982) , 215 - 238 .
• G A J Rogers, Locke, Newton and the Enlightenment, Vistas Astronom. 22 (4) (1978) , 471 - 476 .
• L Rosenfeld, Newton and the law of gravitation, Arch. History Exact Sci. 2 (1964 / 1965) , 365 - 386 .
• R Rynasiewicz, By their properties, causes and effects : Newton's scholium on time, space, place and motion. II. The context, Stud. Hist. Philos. Sci. 26 (2) (1995) , 295 - 321 .
• R Rynasiewicz, By their properties, causes and effects : Newton's scholium on time, space, place and motion. I. The text, Stud. Hist. Philos. Sci. 26 (1) (1995) , 133 - 153 .
• C J Scriba, Erträge der Newton - Forschung, Sudhoffs Arch. 79 (2) (1995) , 150 - 164 .
• A E Shapiro, Beyond the dating game : watermark clusters and the composition of Newton's ' Opticks', in The investigation of difficult things ( Cambridge, 1992) , 181 - 227 .
• A E Shapiro, Huygens' 'Traité de la lumière' and Newton's 'Opticks' : pursuing and eschewing hypotheses, Notes and Records Roy. Soc. London 43 (2) (1989) , 223 - 247 .
• A E Shapiro, The evolving structure of Newton's theory of white light and color, Isis 71 (257) (1980) , 211 - 235 .
• A E Shapiro, Newton's 'achromatic' dispersion law : theoretical background and experimental evidence, Arch. Hist. Exact Sci. 21 (2) (1979 / 80) , 91 - 128 .
• S Di Sieno and M Galuzzi, Section V of the first book of the 'Principia'. Newton and the 'problem of Pappus' ( Italian ) , Arch. Internat. Hist. Sci. 39 (122) (1989) , 51 - 68 .
• D L Simms and P L Hinkley, Brighter than how many suns? Sir Isaac Newton's burning mirror, Notes and Records Roy. Soc. London 43 (1) (1989) , 31 - 51 .
• R Sokolowski, Idealization in Newton's physics, in Newton and the new direction in science ( Vatican City, 1988) , 65 - 72 .
• G Solinas, Newton and Buffon. Newton and the Enlightenment, Vistas Astronom. 22 (4) (1978) , 431 - 439 .
• S K Stein, Exactly how did Newton deal with his planets?, Math. Intelligencer 18 (2) (1996) , 6 - 11 .
• F Steinle, Was ist Masse? Newtons Begriff der Materiemenge, Philos. Natur. 29 (1) (1992) , 94 - 117 .
• L Stewart, Seeing through the scholium : religion and reading Newton in the eighteenth century, Hist. Sci. 34 (104 , 2) (1996) , 123 - 165 .
• E W Strong, Newton's 'mathematical way', J. Hist. Ideas 12 (1951) , 90 - 110 .
• J Such, Newton's fields of study and methodological principia, in Isaac Newton's 'Philosophiae naturalis principia mathematica' ( Singapore, 1988) , 113 - 125 .
• J Sysak, Coleridge's construction of Newton, Ann. of Sci. 50 (1) (1993) , 59 - 81 .
• V Szebehely, Sir Isaac Newton and modern celestial mechanics, Acad. Roy. Belg. Bull. Cl. Sci. (5) 72 (4) (1986) , 220 - 228 .
• F J Tipler, The sensorium of God : Newton and absolute space, in Newton and the new direction in science ( Vatican City, 1988) , 215 - 228 .
• D Topper, Newton on the number of colours in the spectrum, Stud. Hist. Philos. Sci. 21 (2) (1990) , 269 - 279 .
• B Tucha'nska, Newton's discovery of gravity, in Newton and the new direction in science ( Vatican City, 1988) , 45 - 53 .
• I A Tyulina, On the foundations of Newtonian mechanics ( on the 300 th anniversary of Newton's 'Principia' ) ( Russian ) , Istor. Metodol. Estestv. Nauk 36 (1989) , 184 - 196 .
• S R Valluri, C Wilson and W Harper, Newton's apsidal precession theorem and eccentric orbits, J. Hist. Astronom. 28 (1) (1997) , 13 - 27 .
• A C S van Heel, Newton's work on geometrical optical aberrations, Nature 171 (1953) , 305 - 306 .
• S I Vavilov, I Newton's 'Lectures on Optics' ( Russian ) , Akad. Nauk SSSR. Trudy Inst. Istorii Estestvoznaniya 1 (1947) , 315 - 326 .
• C Vilain, La proportionnalité de la masse et du poids dans la dynamique newtonienne, Rev. Histoire Sci. 47 (3 - 4) (1994) , 435 - 473 .
• C Vilain, Newton et le modèle mécaniste de la réfraction, Rev. Histoire Sci. 40 (3 - 4) (1987) , 311 - 324 .
• V Vita, The theory of conics in Newton's 'Principia' ( Italian ) , Archimede 30 (3) (1978) , 130 - 141 .
• Th von Kármán, Isaac Newton and aerodynamics, J. Aeronaut. Sci. 9 (1942) , 521 - 522 .
• O U Vonwiller, Galileo and Newton : their times and ours, J. Proc. Roy. Soc. New South Wales 76 (1943) , 316 - 328 .
• C B Waff, Isaac Newton, the motion of the lunar apogee, and the establishment of the inverse square law, Vistas Astronom. 20 (1 - 2) (1976) , 99 - 103 .
• W A Wallace, Newton's early writings : beginnings of a new direction, in Newton and the new direction in science ( Vatican City, 1988) , 23 - 44 .
• R Weinstock, Newton's 'Principia' and inverse-square orbits : the flaw reexamined, Historia Math. 19 (1) (1992) , 60 - 70 .
• R Weinstock, Long-buried dismantling of a centuries-old myth : Newton's 'Principia' and inverse-square orbits, Amer. J. Phys. 57 (9) (1989) , 846 - 849 .
• R Weinstock, Newton's 'Principia' and the external gravitational field of a spherically symmetric mass distribution, Amer. J. Phys. 52 (10) (1984) , 883 - 890 .
• R Weinstock, Dismantling a centuries-old myth : Newton's 'Principia' and inverse-square orbits, Amer. J. Phys. 50 (7) (1982) , 610 - 617 .
• R S Westfall, Technical Newton : Reviews of five books on Newton's dynamics, Isis 87 (4) (1996) , 701 - 706 .
• R S Westfall, The achievement of Isaac Newton : an essay on the occasion of the three hundredth anniversary of the 'Principia', Math. Intelligencer 9 (4) (1987) , 45 - 49 .
• R S Westfall, Newton and the acceleration of gravity, Arch. Hist. Exact Sci. 35 (3) (1986) , 255 - 272 .
• R S Westfall, Newton's marvelous years of discovery and their aftermath : myth versus manuscript, Isis 71 (256) (1980) , 109 - 121 .
• R S Westfall, A note on Newton's demonstration of motion in ellipses, Arch. Internat. Histoire Sci. 22 (86 - 87) (1969) , 51 - 60 .
• R S Westfall, Huygens' rings and Newton's rings : Periodicity and seventeenth century optics, Ratio 10 (1968) , 64 - 77 .
• D T Whiteside, The mathematical principles underlying Newton's Principia, Journal for the History of Astronomy 1 (1970) , 118 - 119 .
• D T Whiteside, How forceful has a force proof to be? Newton's 'Principia', Book 1 : Corollary 1 to Propositions 11 - 13 , Physis Riv. Internaz. Storia Sci. ( N.S. ) 28 (3) (1991) , 727 - 749 .
• D T Whiteside, Newton the mathematician, in Contemporary Newtonian research ( Dordrecht-Boston, Mass., 1982) , 109 - 127 .
• D T Whiteside, Kepler, Newton and Flamsteed on refraction through a 'regular aire' : the mathematical and the practical, Centaurus 24 (1980) , 288 - 315 .
• D T Whiteside, Newton and dynamics, Bull. Inst. Math. Appl. 13 (9 - 10) (1977) , 214 - 220 .
• D T Whiteside, Newton's lunar theory : from high hope to disenchantment, Vistas Astronom. 19 (4) (1975 / 76) , 317 - 328 .
• D T Whiteside, The mathematical principles underlying Newton's 'Principia mathematica', J. Hist. Astronom. 1 (2) (1970) , 116 - 138 .
• D T Whiteside, Before the 'Principia' : the maturing of Newton's thoughts on dynamical astronomy, 1664 - 1684 , J. Hist. Astronom. 1 (1) (1970) , 5 - 19 .
• G J Whitrow, Newton's role in the history of mathematics, Notes and Records Roy. Soc. London 43 (1) (1989) , 71 - 92 .
• E T Whittaker, Aristotle, Newton, Einstein, Proc. Roy. Soc. Edinburgh Sect. A. 61 (1942) , 231 - 246 .
• E T Whittaker, Aristotle, Newton, Einstein, Philos. Mag. (7) 34 (1943) , 266 - 280 .
• A J Wojtcuk, Newton's childhood, in Newton and the new direction in science ( Vatican City, 1988) , 261 - 264 .
• H Wussing, Isaac Newton - Leben und Werk, NTM Schr. Geschichte Natur. Tech. Medizin 15 (1) (1978) , 71 - 80 .
• I M Yaglom, Why was higher mathematics simultaneously discovered by Newton and Leibniz? ( Russian ) , in Number and thought 6 ( Moscow, 1983) , 99 - 125 .
• K N Yan, A re-examination into Newton's definition of mass and Mach's criticism, Historia Sci. 40 (1990) , 29 - 40 .
• K N Yan, On Isaac Newton's ideas of gravitation and God, Historia Sci. 34 (1988) , 43 - 56 .
• A P Youschkevitch, Newton and the mathematical natural sciences ( on the 300 th anniversary of the 'Principia' ) ( Russian ) , Mat. v Shkole (1) (1988) , 64 - 67 .
• A P Youschkevitch, Comparaison des conceptions de Leibniz et de Newton sur le calcul infinitésimal, in Leibniz in Paris (1672 - 1676) ( Wiesbaden, 1978) , 69 - 80 .
• M Yurkina, Newton's 'Principia' and the origin of the modern theory of the shape of the Earth ( Russian ) , Istor.-Astronom. Issled. 20 (1988) , 56 - 63 .

Additional Resources ( show )

Other pages about Isaac Newton:

• John Maynard Keynes' Newton the Man
• Newton's Arian beliefs
• Flamsteed v Newton
• Charles Bossut on Leibniz and Newton
• Newton's Principia Preface
• John Collins meets Isaac Newton
• Julian and Gregorian calendars
• Newton-Raphson method
• Woolsthorpe Manor
• The title page of Philosophiae naturalis principia mathematica ( The Principia 1687)
• The title page of Analysis per quantitatum series fluxiones (1711)
• Isaac Newton by his contemporaries
• Multiple entries in The Mathematical Gazetteer of the British Isles ,
• Astronomy: The Reaches of the Milky Way
• Astronomy: The Dynamics of the Solar System
• Henry Taylor on Isaac Newton
• Miller's postage stamps
• Heinz Klaus Strick biography

Other websites about Isaac Newton:

• Dictionary of Scientific Biography
• Dictionary of National Biography
• Encyclopaedia Britannica
• The Newton Project ( UK )
• The Galileo Project
• G Don Allen
• Sci Hi blog
• A Google doodle
• Kevin Brown ( More about Newton's birthday )
• Mathematical Genealogy Project
• MathSciNet Author profile

Honours ( show )

Honours awarded to Isaac Newton

• Lucasian Professor 1669
• Fellow of the Royal Society 1672
• President of the Royal Society 1703 - 1727
• Lunar features Crater Newton
• Paris street names Rue Newton (16 th Arrondissement )
• Popular biographies list Number 3
• Google doodle 2010

Cross-references ( show )

• History Topics: A brief history of cosmology
• History Topics: A chronology of π
• History Topics: A history of the calculus
• History Topics: A history of time: 20 th century time
• History Topics: A history of time: Classical time
• History Topics: A mathematical walk around Bologna
• History Topics: An overview of Indian mathematics
• History Topics: An overview of the history of mathematics
• History Topics: Christianity and the Mathematical Sciences - the Heliocentric Hypothesis
• History Topics: Elliptic functions and integrals
• History Topics: English attack on the Longitude Problem
• History Topics: Fermat's last theorem
• History Topics: General relativity
• History Topics: Greek astronomy
• History Topics: Infinity
• History Topics: Light through the ages: Ancient Greece to Maxwell
• History Topics: London Coffee houses and mathematics
• History Topics: Longitude and the Académie Royale
• History Topics: Mathematical discovery of planets
• History Topics: Mathematics and Architecture
• History Topics: Mathematics and the physical world
• History Topics: Mathematics in St Andrews to 1700
• History Topics: Newton's bucket
• History Topics: Orbits and gravitation
• History Topics: Overview of Chinese mathematics
• History Topics: Science in the 17 th century: From Europe to St Andrews
• History Topics: Special relativity
• History Topics: The Bakhshali manuscript
• History Topics: The Berlin Academy and forgery
• History Topics: The brachistochrone problem
• History Topics: The development of the 'black hole' concept
• History Topics: The mathematician and the forger
• History Topics: Theories of gravitation
• History Topics: Weather forecasting
• Famous Curves: Cartesian Oval
• Famous Curves: Cissoid of Diocles
• Famous Curves: Conchoid
• Famous Curves: Cycloid
• Famous Curves: Epicycloid
• Famous Curves: Epitrochoid
• Famous Curves: Hypocycloid
• Famous Curves: Hypotrochoid
• Famous Curves: Kappa Curve
• Famous Curves: Lissajous Curves
• Famous Curves: Newton's Diverging Parabolas
• Famous Curves: Parabola
• Famous Curves: Serpentine
• Famous Curves: Trident of Newton
• Student Projects: Indian Mathematics - Redressing the balance: Chapter 11
• Student Projects: Indian Mathematics - Redressing the balance: Chapter 13
• Student Projects: Indian Mathematics - Redressing the balance: Chapter 14
• Student Projects: Indian Mathematics - Redressing the balance: Chapter 15
• Student Projects: Indian Mathematics - Redressing the balance: Chapter 18
• Student Projects: James Clerk Maxwell - The Great Unknown: Chapter 1
• Student Projects: James Clerk Maxwell - The Great Unknown: Chapter 5
• Student Projects: James Clerk Maxwell - The Great Unknown: Chapter 8
• Other: 12th June
• Other: 13th May
• Other: 15th February
• Other: 15th January
• Other: 1897 ICM - Zurich
• Other: 1904 ICM - Heidelberg
• Other: 1908 ICM - Rome
• Other: 1912 ICM - Cambridge
• Other: 1924 ICM - Toronto
• Other: 1928 ICM - Bologna
• Other: 1932 ICM - Zurich
• Other: 1950 ICM - Cambridge USA
• Other: 19th February
• Other: 2009 Most popular biographies
• Other: 25th December
• Other: 26th January
• Other: 28th September
• Other: 2nd June
• Other: 2nd October
• Other: 30th November
• Other: 31st July
• Other: 3rd November
• Other: 4th September
• Other: 5th July
• Other: 6th December
• Other: 6th January
• Other: Cambridge Colleges
• Other: Cambridge Individuals
• Other: Cambridge professorships
• Other: Earliest Known Uses of Some of the Words of Mathematics (A)
• Other: Earliest Known Uses of Some of the Words of Mathematics (C)
• Other: Earliest Known Uses of Some of the Words of Mathematics (D)
• Other: Earliest Known Uses of Some of the Words of Mathematics (E)
• Other: Earliest Known Uses of Some of the Words of Mathematics (F)
• Other: Earliest Known Uses of Some of the Words of Mathematics (G)
• Other: Earliest Known Uses of Some of the Words of Mathematics (H)
• Other: Earliest Known Uses of Some of the Words of Mathematics (I)
• Other: Earliest Known Uses of Some of the Words of Mathematics (L)
• Other: Earliest Known Uses of Some of the Words of Mathematics (M)
• Other: Earliest Known Uses of Some of the Words of Mathematics (N)
• Other: Earliest Known Uses of Some of the Words of Mathematics (O)
• Other: Earliest Known Uses of Some of the Words of Mathematics (P)
• Other: Earliest Known Uses of Some of the Words of Mathematics (Q)
• Other: Earliest Known Uses of Some of the Words of Mathematics (R)
• Other: Earliest Known Uses of Some of the Words of Mathematics (S)
• Other: Earliest Known Uses of Some of the Words of Mathematics (T)
• Other: Earliest Uses of Symbols of Calculus
• Other: Earliest Uses of Symbols of Operation
• Other: Jeff Miller's postage stamps
• Other: London Learned Societies
• Other: London Miscellaneous
• Other: London Museums
• Other: London Schools
• Other: London Scientific Institutions
• Other: London individuals A-C
• Other: London individuals H-M
• Other: London individuals N-R
• Other: London individuals S-Z
• Other: Most popular biographies – 2024
• Other: On Growth and Form
• Other: Other Institutions in central London
• Other: Other London Institutions outside the centre
• Other: Oxford Institutions and Colleges
• Other: Oxford individuals
• Other: Oxford professorships
• Other: Popular biographies 2018
• Other: The Dynamics of the Solar System
• Other: The Reaches of the Milky Way

Sir Isaac Newton biography: Inventions, laws and quotes

A short history of Sir Isaac Newton, the mathematician and physicist that helped invent and explain some of the most fundamental laws of science.

Isaac Newton's early life

• Laws of motion

Isaac Newton's apple

• Inventions and discoveries

Additional resources

Bibliography.

Sir Isaac Newton contributed significantly to the field of science over his lifetime. He invented calculus and provided a clear understanding of optics. But his most significant work had to do with forces, and specifically with the development of a universal law of gravitation and his laws of motion . 

Isaac Newton was born on Christmas Day to a poor farming family in Woolsthorpe, England, in 1642. At the time of Newton's birth England used the Julian calendar, however, when England adopted the Gregorian calendar in 1752, his birthday became 4th January 1643. 

Isaac Newton arrived in the world only a few months after his father, Isaac Newton Sr, had died. "The boy expected to live managing the farm in the place of the father he had never known," wrote James Gleick in "Isaac Newton" ( Vintage, 2004 ). 

However, when it became clear a farming life was not for him, Newton attended Trinity College in Cambridge, England. "He did not know what he wanted to be or do, but it was not tend sheep or follow the plough and the dung cart," wrote Gleick. While there, he took an interest in mathematics, optics, physics, and astronomy . 

After his graduation, he began to teach at the college and was appointed as the second Lucasian Chair there. Today, the chair is considered the most renowned academic chair in the world, held by the likes of Charles Babbage and Stephen Hawking .

In 1689, Newton was elected as a member of parliament for the university. In 1703, he was elected as president of the Royal Society, a fellowship of scientists that still exists today. He was knighted by Queen Anne in 1705. He never married.

What are Isaac Newton's laws of motion?

Newton's most famous work came with the publication of his " Philosophiae Naturalis Principia Mathematica " ("Mathematical Principles of Natural Philosophy"), generally called Principia. In it, he determined the three laws of motion for the universe .

Newton's first law describes how objects move at the same velocity unless an outside force acts upon them. (A force is something that causes or changes motion.) Thus, an object sitting on a table remains on the table until a force — the push of a hand, or gravity — acts upon it. Similarly, an object travels at the same speed unless it interacts with another force, such as friction.

His second law of motion provided a calculation for how forces interact. The law states that a force is equal to the change in the momentum (mass multiplied by velocity) per change in time. Therefore, when more force is applied to an object, its acceleration also increases, but when the mass of the object increases and the force remains constant, its acceleration decreases.

Newton's third law states that for every action in nature, there is an equal and opposite reaction. If one body applies a force on a second, then the second body exerts a force of the same strength on the first, in the opposite direction. 

From all of this, Newton calculated the universal law of gravitation. He found that as two bodies move farther away from one another, the gravitational attraction between them decreases by the inverse of the square of the distance. Thus, if the objects are twice as far apart, the gravitational force is only a fourth as strong; if they are three times as far apart, it is only a ninth of its previous power.

These laws helped scientists understand more about the motions of planets in the solar system , and of the moon around Earth.

Related: What makes Newton's laws work? Here's the simple trick.

A popular myth tells of an apple falling from a tree in Newton's garden, which brought Newton to an understanding of forces, particularly gravity. Whether the incident actually happened is unknown, but historians doubt the event — if it occurred — was the driving force in Newton's thought process.

The myth tells of Isaac Newton having returned to his family farm in Woolsthorpe, escaping Cambridge for a short time as it was dealing with a plague outbreak. As he sat in the farm's orchard, an apple fell from one of the trees (in some tellings it hit Newton on the head). Watching this happen, Newton began to consider the forces that meant the apple always fell directly towards the ground, beginning his examination of gravity.

One of the reasons that this story gained a foothold in popular understanding is that it is an anecdote Newton himself seems to have shared. "Toward the end of his life, Newton told the apple anecdote around four times, although it only became well known in the nineteenth century," wrote Patricia Fara, a historian of science at the University of Cambridge, in a chapter of " Newton's Apple and Other Myths about Science " (Harvard University Press, 2020).

However, it would be at least 20 years before Newton published his theories on gravity. It seems more likely that Newton used the story as a means of connecting the concept of gravity's impact on objects on Earth with its impact on objects in space for his contemporary audience.

The apple tree in question — known as the "Flower of Kent" — still blooms in the orchard of Woolsthorpe Manor, and is now a popular tourist attraction.  

Isaac Newton's inventions and discoveries

— Famous astronomers: How these scientists shaped astronomy  

— What is Astrophysics?

— Physicists crack unsolvable three-body problem using drunkard's walk  

While a student, Newton was forced to take a two-year hiatus when plague closed Trinity College. At home, he continued to work with optics, using a prism to separate white light, and became the first person to argue that white light was a mixture of many types of rays, rather than a single entity. He continued working with light and color over the next few years and published his findings in " Opticks " in 1704.

Disturbed by the problems with telescopes at the time, he invented the reflecting telescope, grinding the mirror and building the tube himself. Relying on a mirror rather than lenses, the telescope presented a sharper image than refracting telescopes at the time. Modern techniques have reduced the problems caused by lenses, but large telescopes such as the James Webb Space Telescope use mirrors. 

As a student, Newton studied the most advanced mathematical texts of his time. While on hiatus, he continued to study mathematics, laying the ground for differential and integral calculus. He united many techniques that had previously been considered separately, such as finding areas, tangents, and the lengths of curves. He wrote De Methodis Serierum et Fluxionum in 1671 but was unable to find a publisher.

Newton also established a cohesive scientific method, to be used across disciplines. Previous explorations of science varied depending on the field. Newton established a set format for experimentation still used today.

However, not all of Newton's ideas were quite as revolutionary. In P rincipia, Newton describes how rarefied vapor from comet tails is pulled into Earth's gravitational grasp and enables the movements of the planet's fluids along with the "most subtle and useful part of our air, and so much required to sustain the life of all things with us." 

Isaac Newton quotes

"Amicus Plato amicus Aristoteles magis amica verita."

(Plato is my friend, Aristotle is my friend, but my greatest friend is truth.)

—Written in the margin of a notebook while a student at Cambridge. In Richard S. Westfall, Never at Rest (1980), 89.

"Genius is patience."

—The Homiletic Review, Vol. 83-84 (1922), Vol. 84, 290.

"If I have seen further it is by standing on the shoulders of giants."

—Letter to Robert Hooke (5 Feb 1675-6).In H. W. Turnbull (ed.), The Correspondence of Isaac Newton, 1, 1661-1675 (1959), Vol. 1, 416.

"I see I have made my self a slave to Philosophy."

—Letter to Henry Oldenburg (18 Nov 1676). In H. W. Turnbull (ed.), The Correspondence of Isaac Newton, 1676-1687 (1960), Vol. 2, 182.

"I do not know what I may appear to the world, but to myself I seem to have been only like a boy playing on the seashore, and diverting myself in now and then finding a smoother pebble or a prettier shell than ordinary, whilst the great ocean of truth lay all undiscovered before me."

—First reported in Joseph Spence, Anecdotes, Observations and Characters, of Books and Men (1820), Vol. 1 of 1966 edn, sect. 1259, p. 462

"To any action there is always an opposite and equal reaction; in other words, the actions of two bodies upon each other are always equal and always opposite in direction."

— The Principia: Mathematical Principles of Natural Philosophy (1687)

"Truth is ever to be found in simplicity, and not in the multiplicity and confusion of things."

—'Fragments from a Treatise on Revelation". In Frank E. Manuel, The Religion of Isaac Newton (1974), 120.

How did Sir Isaac Newton die?

Newton died in 1727 during his sleep at the age of 84. Although the cause of death is unknown, a 1979 study published by Newton's own Royal Society suggests mercury poisoning may have contributed to the decline of his physical and mental health. During the exhumation of his body, large amounts of mercury were found in the scientist's system, likely due to his work with alchemy. Newton conducted several experiments to convert base metals, such as mercury and copper into precious metals, such as gold and silver. 

"In 1693 Newton suffered from insomnia and poor digestion; and he also wrote irrational letters to friends. Although most scholars have attributed Newton's breakdown to psychological factors, it is possible that mercury poisoning may have been the principal cause," wrote L. W. Johnson and M. L. Wolbarsht " Mercury Poisoning: A probable cause of Isaac Newton's physical and mental ills: Notes and Records of the Royal Society of London Vol. 34. No. 1. " .

After his death, his body was moved to a more prominent place in Westminster Abbey. His white and grey marble monument stands in the nave of the Abbey's choir screen and boasts sculptures of Newton lounging surrounded by children using the many instruments, such as telescopes, associated with Newton's work. The inscription on the monument — originally written in Latin — reads: 

" Here is buried Isaac Newton, Knight, who by a strength of mind almost divine, and mathematical principles peculiarly his own, explored the course and figures of the planets, the paths of comets, the tides of the sea, the dissimilarities in rays of light, and, what no other scholar has previously imagined, the properties of the colours thus produced. Diligent, sagacious and faithful, in his expositions of nature, antiquity and the holy Scriptures, he vindicated by his philosophy the majesty of God mighty and good, and expressed the simplicity of the Gospel in his manners. Mortals rejoice that there has existed such and so great an ornament of the human race! He was born on 25th December 1642, and died on 20th March 1726. " The date of his death on his monument is given in the Julian calendar. 

If you want to learn more about the impact of this celebrated scientist, then you should read about how Isaac Newton Changed the World . If you're wondering whether Newton's second law of motion works in space then an Astronaut has tested the theory out.

"Isaac Newton" by James Gleick (Vintage, 2004 )

" Mercury Poisoning: A probable cause of Isaac Newton's physical and mental ills: Notes and Records of the Royal Society of London Vol. 34. No. 1. " by L. W. Johnson and M. L. Wolbarsht (July 1979)

" The Mathematical Principles of Natural Philosophy " by Isaac Newton (Flame Tree Collections, 2020)

" Newton's Apple and Other Myths about Science " edited by Ronald L. Numbers and Kostas Kampourakis (Harvard University Press, 2020)

" Life After Gravity: Isaac Newton's London Career " by Patricia Fara (Oxford University Press, 2021)

"Isaac Newton" Stanford Encyclopedia of Philosophy (2007)

"Isaac Newton" University of St Andrews (2000)

"Sir Isaac Newton" Westminster Abbey (2023)

Join our Space Forums to keep talking space on the latest missions, night sky and more! And if you have a news tip, correction or comment, let us know at: [email protected].

Get the Space.com Newsletter

Breaking space news, the latest updates on rocket launches, skywatching events and more!

Nola Taylor Tillman is a contributing writer for Space.com. She loves all things space and astronomy-related, and enjoys the opportunity to learn more. She has a Bachelor’s degree in English and Astrophysics from Agnes Scott college and served as an intern at Sky & Telescope magazine. In her free time, she homeschools her four children. Follow her on Twitter at @NolaTRedd

Hubble Telescope sees 'stellar volcano' erupt in amazing colors (video, photo)

US Space Force awards SpaceX $730 million to launch at least 9 national-security missions

SpaceX rolls out Super Heavy rocket for Starship Flight 6 test launch (photos)

Most Popular

• 2 'Futurama' creator Matt Groening says 'great episodes in the works' for upcoming Season 13
• 3 Headless 'Halloween comet' could already be doomed
• 4 Mini '2001' monoliths fly into space on first flight of Blue Origin's 2nd crew ship
• 5 Spooky fireball blazes across Lake Erie a week before Halloween (video)

Sir Isaac Newton Online

Why Isaac Newton is the Greatest Physicist of All Time An intro to Newton’s discoveries – all before the age of 26! Isaac Newton Videos

Most famous for discovering the laws of gravity and motion, plus inventing physics and calculus, Isaac Newton is generally regarded as the most original and influential theorist in the history of science.

Sir isaac newton biography, sir isaac newton facts.

Quick and fun facts about Isaac Newton from basic to advanced to just plain odd.

POPULAR: What Was Isaac Newton’s IQ?

Isaac Newton’s Discoveries & Inventions

Law of gravity, laws of motion.

Isaac Newton discovered three laws of motion that became the basis of modern physics.

Quotes by Isaac Newton

Tact is the art of making a point without an enemy.

To every action there is always opposed an equal reaction.

How Isaac Newton Changed Our World

One of the most influential scientists in history, Sir Isaac Newton ’s contributions to the fields of physics, mathematics, astronomy and chemistry helped usher in the Scientific Revolution. And while the long-told tale of an apple dropping on his learned head is likely apocryphal, his contributions changed the way we see and understand the world around us.

He created the modern telescope

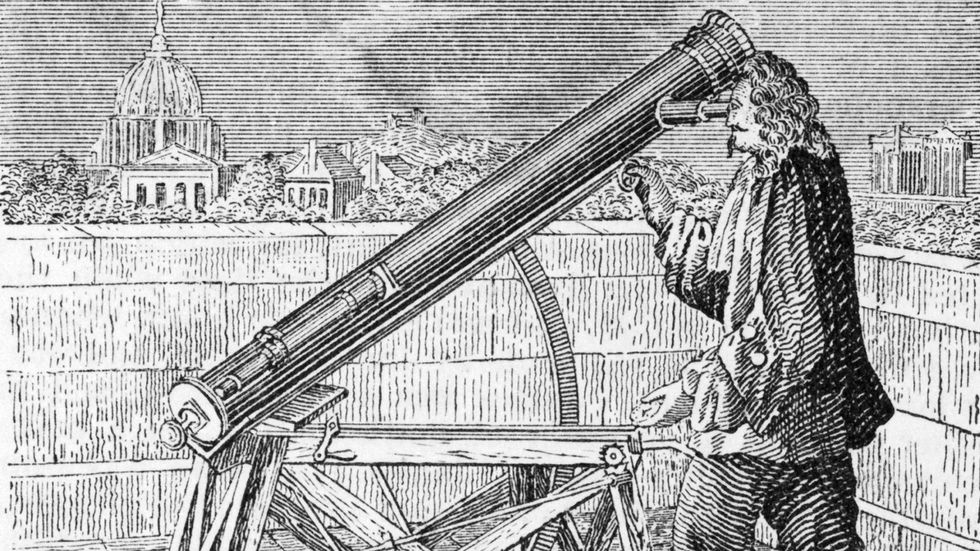
Before Newton, standard telescopes provided magnification, but with drawbacks. Known as refracting telescopes, they used glass lenses that changed the direction of different colors at different angles. This caused “chromatic aberrations,” or fuzzy, out-of-focus areas around objects being viewed through the telescope.

After much tinkering and testing, including grinding his own lenses, Newton found a solution. He replaced the refracting lenses with mirrored ones, including a large, concave mirror to show the primary image and a smaller, flat, reflecting one, to display that image to the eye. Newton’s new “reflecting telescope” was more powerful than previous versions, and because he used the small mirror to bounce the image to the eye, he could build a much smaller, more practical telescope. In fact, his first model, which he built in 1668 and donated to England’s Royal Society, was just six inches long (some 10 times smaller than other telescopes of the era), but could magnify objects by 40x.

Newton’s simple telescope design is still used today, by both backyard astronomers and NASA scientists.

Newton helped develop spectral analysis

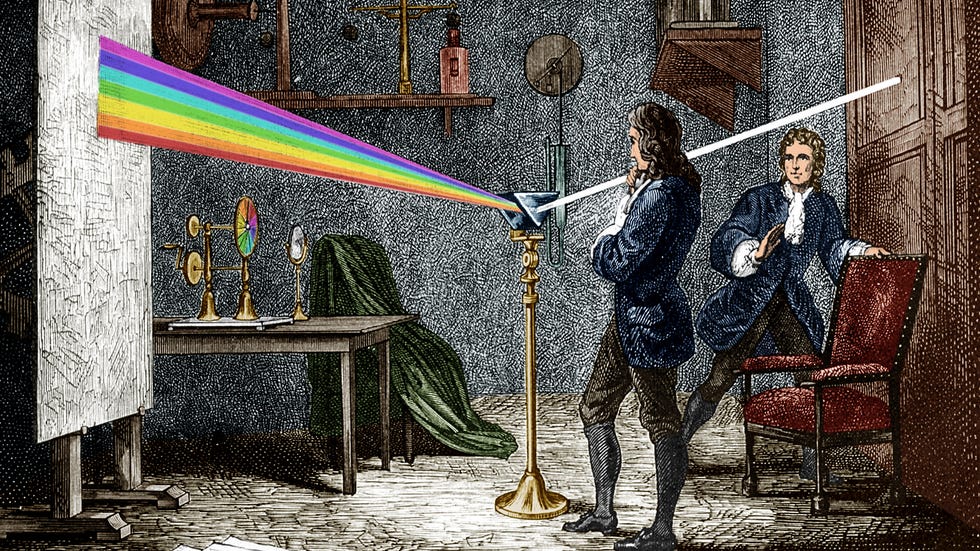
The next time you look up at a rainbow in the sky, you can thank Newton for helping us first understand and identify its seven colors. He began working on his studies of light and color even before creating the reflecting telescope, although he presented much of his evidence several years later, in his 1704 book, Opticks .

Before Newton, scientists primarily adhered to ancient theories on color, including those of Aristotle , who believed that all colors came from lightness (white) and darkness (black). Some even believed that the colors of the rainbow were formed by rainwater that colored the sky’s rays. Newton disagreed. He performed a seemingly endless series of experiments to prove his theories.

Working in his darkened room, he directed white light through a crystal prism on a wall, which separated into the seven colors we now know as the color spectrum (red, orange, yellow, green, blue, indigo, and violet). Scientists already knew many of these colors existed, but they believed that the prism itself transformed white light into these colors. But when Newton refracted these same colors back onto another prism, they formed into a white light, proving that white light (and sunlight) was actually a combination of all the colors of the rainbow.

Newton’s laws of motion laid the groundwork for classical mechanics

In 1687, Newton published one of the most important scientific books in history, the Philosophiae Naturalis Principia Mathematica , commonly known as the Principa . It was in this work that he first laid out his three laws of motion.

The law of inertia states that at rest or in motion will remain at rest or in motion unless it’s acted upon by an external force. So, with this law, Newton helps us explain why a car will stop when it hits a wall, but the human bodies within the car will keep moving at the same, constant speed they had been until the bodies hit an external force, like a dashboard or airbag. It also explains why an object thrown in space is likely to continue at the same speed on the same path for infinity unless it comes into another object that exerts force to slow it down or change direction.

You can see an example of his second law of acceleration when you ride a bicycle. In his equation that force equals mass times acceleration, or F=ma , your pedaling of a bicycle creates the force necessary to accelerate. Newton’s law also explains why larger or heavier objects require more force to move or alter them, and why hitting a small object with a baseball bat would produce more damage than hitting a large object with that same bat.

His third law of action and reaction creates a simple symmetry to the understanding of the world around us: For every action, there is an equal and opposite reaction. When you sit in a chair, you are exerting force down upon the chair, but the chair is exerting equal force to keep you upright. And when a rocket is launched into space, it’s thanks to the backward force of the rocket upon gas and the forward thrust of the gas on the rocket.

He created the law of universal gravitation and calculus

The Principa also contained some of Newton’s first published works on the motion of the planets and gravity. According to a popular legend, a young Newton was sitting beneath a tree on his family’s farm when the falling of an apple inspired one of his most famous theories. It’s impossible to know if this is true (and Newton himself only began telling the story as an older man), but is a helpful story to explain the science behind gravity. It also remained the basis of classical mechanics until Albert Einstein’s theory of relativity.

Newton worked out that if the force of gravity pulled the apple from the tree, then it was also possible for gravity to exert its pull on objects much, much further away. Newton’s theory helped prove that all objects, as small as an apple and as large as a planet, are subject to gravity. Gravity helped keep the planets rotating around the sun and creates the ebbs and flows of rivers and tides. Newton’s law also states that larger bodies with heavier masses exert more gravitational pull, which is why those who walked on the much smaller moon experienced a sense of weightlessness, as it had a smaller gravitational pull.

To help explain his theories of gravity and motion, Newton helped create a new, specialized form of mathematics. Originally known as “fluxions,” and now calculus, it charted the constantly changing and variable state of nature (like force and acceleration), in a way that existing algebra and geometry could not. Calculus may have been the bane of many a high school and college student, but it has proved invaluable to centuries of mathematicians, engineers and scientists.

Famous Mathematicians

Did This Newly Found Compass Belong to Copernicus?

The Solar Eclipse That Made Albert Einstein a Star

Archimedes: The Mathematician Who Discovered Pi

Benjamin Banneker

22 Famous Scientists You Should Know

Charles Babbage

Blaise Pascal

Leonhard Euler

Ada Lovelace

Valerie Thomas

Biographies for Kids

Isaac newton.

• Occupation: Scientist, mathematician, and astronomer
• Born: January 4, 1643 in Woolsthorpe, England
• Died: March 31, 1727 in London, England
• Best known for: Defining the three laws of motion and universal gravitation

• Gravity - Newton is probably most famous for discovering gravity. Outlined in the Principia, his theory about gravity helped to explain the movements of the planets and the Sun. This theory is known today as Newton's law of universal gravitation.
• Laws of Motion - Newton's laws of motion were three fundamental laws of physics that laid the foundation for classical mechanics.
• Calculus - Newton invented a whole new type of mathematics which he called "fluxions." Today we call this math calculus and it is an important type of math used in advanced engineering and science.
• Reflecting Telescope - In 1668 Newton invented the reflecting telescope . This type of telescope uses mirrors to reflect light and form an image. Nearly all of the major telescopes used in astronomy today are reflecting telescopes.
• He studied many classic philosophers and astronomers such as Aristotle, Copernicus, Johannes Kepler, Rene Descartes, and Galileo.
• Legend has it that Newton got his inspiration for gravity when he saw an apple fall from a tree on his farm.
• He wrote his thoughts down in the Principia at the urging of his friend (and famous astronomer) Edmond Halley. Halley even paid for the book's publication.
• He once said of his own work "If I have seen further than others, it is by standing upon the shoulders of giants."
• Listen to a recorded reading of this page: